Electric Machinery--Polyphase Induction Machines

The objective of this section is to study the behavior of polyphase induction machines. Our analysis will begin with the development of single-phase equivalent circuits, the general form of which is suggested by the similarity of an induction machine to a transformer. These equivalent circuits can be used to study the electromechanical characteristics of an induction machine as well as the loading presented by the machine on its supply source, whether it is a fixed-frequency source such as a power system or a variable-frequency, variable-voltage motor drive.

1. INTRODUCTION TO POLYPHASE INDUCTION MACHINES

As indicated in Section 4.2.1, an induction motor is one in which alternating current is supplied to the stator directly and to the rotor by induction or transformer action from the stator. As in the synchronous machine, the stator winding is of the type discussed in Section 4.5. When excited from a balanced polyphase source, it will produce a magnetic field in the air gap rotating at synchronous speed as determined by the number of stator poles and the applied stator frequency fe (Eq. 4.41). The rotor of a polyphase induction machine may be one of two types. A wound rotor is built with a polyphase winding similar to, and wound with the same number of poles as, the stator. The terminals of the rotor winding are connected to insulated slip rings mounted on the shaft. Carbon brushes bearing on these rings make the rotor terminals available external to the motor, as shown in the cutaway view in FIG. 1.

Wound-rotor induction machines are relatively uncommon, being found only in a limited number of specialized applications.

FIG. 1 Cutaway view of a three-phase induction motor with a wound rotor and slip rings connected to the three-phase rotor winding. (General Electric Company.)

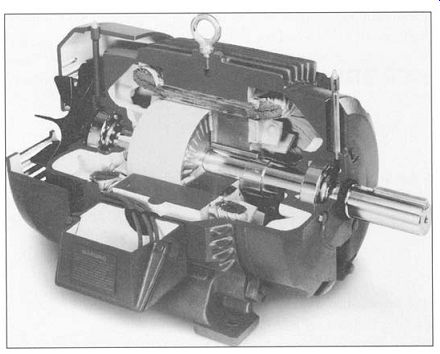
FIG. 2 Cutaway view of a three-phase squirrel-cage motor. The rotor cutaway shows the squirrel-cage laminations. (Rockwell Automation-Reliance Electric.)

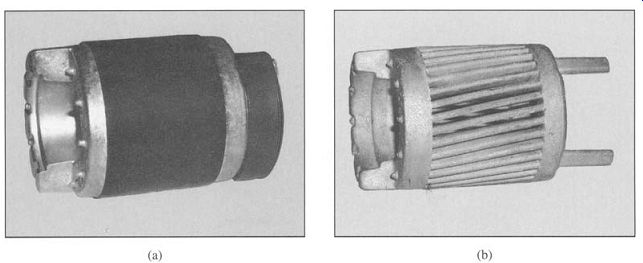
FIG. 3 (a) The rotor of a small squirrel-cage motor. (b) The squirrel-cage structure after the rotor laminations have been chemically etched away. ( Athens Products.)

On the other hand, the polyphase induction motor shown in cutaway in FIG. 2 has a squirrel-cage rotor with a winding consisting of conducting bars embedded in slots in the rotor iron and short-circuited at each end by conducting end rings. The extreme simplicity and ruggedness of the squirrel-cage construction are outstanding advantages of this type of induction motor and make it by far the most commonly used type of motor in sizes ranging from fractional horsepower on up. FIG. 3a shows the rotor of a small squirrel-cage motor while FIG. 3b shows the squirrel cage itself after the rotor laminations have been chemically etched away.

Let us assume that the rotor is turning at the steady speed of n r/min in the same direction as the rotating stator field. Let the synchronous speed of the stator field be ns r/min as given by Eq. 4.41. This difference between synchronous speed and the rotor speed is commonly referred to as the slip of the rotor; in this case the rotor slip is ns n, as measured in r/min. Slip is more usually expressed as a fraction of synchronous speed. The fractional slip s is...

(Eq. 1)

The slip is often expressed in percent, simply equal to 100 percent times the fractional slip of Eq. 1.

The rotor speed in r/min can be expressed in terms of the slip and the synchronous speed as

n --(1 s)ns (Eq. 2)

Similarly, the mechanical angular velocity COm can be expressed in terms of the synchronous angular velocity COs and the slip as...

(Eq. 3)

The relative motion of the stator flux and the rotor conductors induces voltages of frequency fr....

fr = Sfe (Eq. 4)

...called the slip frequency, in the rotor. Thus, the electrical behavior of an induction machine is similar to that of a transformer but with the additional feature of frequency transformation produced by the relative motion of the stator and rotor windings. In fact, a wound-rotor induction machine can be used as a frequency changer.

The rotor terminals of an induction motor are short circuited; by construction in the case of a squirrel-cage motor and externally in the case of a wound-rotor motor.

The rotating air-gap flux induces slip-frequency voltages in the rotor windings. The rotor currents are then determined by the magnitudes of the induced voltages and the rotor impedance at slip frequency. At starting, the rotor is stationary (n = 0), the slip is unity (s = 1), and the rotor frequency equals the stator frequency fe. The field produced by the rotor currents therefore revolves at the same speed as the stator field, and a starting torque results, tending to turn the rotor in the direction of rotation of the stator-inducing field. If this torque is sufficient to overcome the opposition to rotation created by the shaft load, the motor will come up to its operating speed.

The operating speed can never equal the synchronous speed however, since the rotor conductors would then be stationary with respect to the stator field; no current would be induced in them, and hence no torque would be produced.

With the rotor revolving in the same direction of rotation as the stator field, the frequency of the rotor currents is safe and they will produce a rotating flux wave which will rotate at Sns r/min with respect to the rotor in the forward direction. But superimposed on this rotation is the mechanical rotation of the rotor at n r/min. Thus, with respect to the stator, the speed of the flux wave produced by the rotor currents is the sum of these two speeds and equals...

(Eq. 5)

From Eq. 5 we see that the rotor currents produce an air-gap flux wave which rotates at synchronous speed and hence in synchronism with that produced by the stator currents. Because the stator and rotor fields each rotate synchronously, they are stationary with respect to each other and produce a steady torque, thus maintaining rotation of the rotor. Such torque, which exists for any mechanical rotor speed n other than synchronous speed, is called an asynchronous torque.

FIG. 4 shows a typical polyphase squirrel-cage induction motor torque-speed curve. The factors influencing the shape of this curve can be appreciated in terms of the torque equation, Eq. 4.81. Note that the resultant air-gap flux ~sr in this equation is approximately constant when the stator-applied voltage and frequency are constant.

Also, recall that the rotor mmf Fr is proportional to the rotor current Ir. Equation 4.81 can then be expressed in the form...

(Eq. 6)

where K is a constant and r is the angle by which the rotor mmf wave leads the resultant air-gap mmf wave.

FIG. 4 Typical induction-motor torque-speed curve for constant-voltage, constant-frequency operation.

The rotor current is equal to the negative of the voltage induced by the air-gap flux divided by the rotor impedance, both at slip frequency. The minus sign is required because the induced rotor current is in the direction to demagnetize the air-gap flux, whereas the rotor current is defined in Section 4 as being in the direction to magnetize the air gap. Under normal running conditions the slip is small: 2 to 10 percent at full load in most squirrel-cage motors. The rotor frequency (fr = Sfe) therefore is very low (of the order of 1 to 6 Hz in 60-Hz motors). In this range the rotor impedance is largely resistive and hence independent of slip. The rotor-induced voltage, on the other hand, is proportional to slip and leads the resultant air-gap flux by 90 ° . Thus the rotor current is very nearly proportional to the slip, and proportional to and 180 ° out of phase with the rotor voltage. As a result, the rotor-mmf wave lags the resultant air-gap flux by approximately 90 electrical degrees, and therefore sin gr ~ 1.

Approximate proportionality of torque with slip is therefore to be expected in the range where the slip is small. As slip increases, the rotor impedance increases because of the increasing contribution of the rotor leakage inductance. Thus the rotor current is less than proportional to slip. Also the rotor current lags farther behind the induced voltage, and the magnitude of sin ~r decreases.

The result is that the torque increases with increasing slip up to a maximum value and then decreases, as shown in FIG. 4. The maximum torque, or breakdown torque, which is typically a factor of two larger than the rated motor torque, limits the short-time overload capability of the motor.

We will see that the slip at which the peak torque occurs is proportional to the rotor resistance. For squirrel-cage motors this peak-torque slip is relatively small, much as is shown in FIG. 4. Thus, the squirrel-cage motor is substantially a constant-speed motor having a few percent drop in speed from no load to full load. In the case of a wound-rotor motor, the rotor resistance can be increased by inserting external resistance, hence increasing the slip at peak-torque, and thus decreasing the motor speed for a specified value of torque. Since wound-rotor induction machines are larger, more expensive and require significantly more maintenance than squirrel-cage machines, this method of speed control is rarely used, and induction machines driven from constant-frequency sources tend to be limited to essentially constant-speed applications. As we will see in Section 11, use of solid-state, variable-voltage, variable-frequency drive systems makes it possible to readily control the speed of squirrel-cage induction machines and, as a result, they are now widely used in a wide-range of variable-speed applications.

2. CURRENTS AND FLUXES IN POLYPHASE INDUCTION MACHINES

For a coil-wound rotor, the flux-mmf situation can be seen with the aid of FIG. 5.

This sketch shows the development of a simple two-pole, three-phase rotor winding in a two-pole field. It therefore conforms with the restriction that a wound rotor must have the same number of poles as the stator (although the number of phases need not be the same). The rotor flux-density wave is moving to the fight at angular velocity COs and at slip angular velocity SCOs with respect to the rotor winding, which in turn is rotating to the fight at angular velocity (1 s)COs. It is shown in FIG. 5 in the position of maximum instantaneous voltage in phase a.

If the rotor leakage reactance, equal to s cos times the rotor leakage inductance, is very small compared with the rotor resistance (which is typically the case at the small slips corresponding to normal operation), the phase-a current will also be a maximum. As shown in Section 4.5, the rotor-mmf wave will then be centered on phase a; it is so shown in FIG. 5a. The displacement angle, or torque angle, ~r under these conditions is at its optimum value of -90 ° .

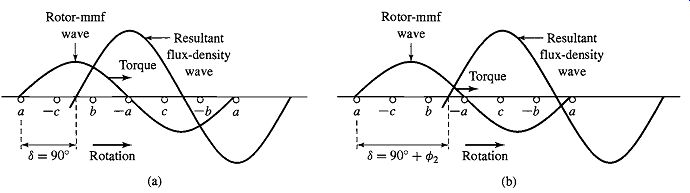
FIG. 5 Developed rotor winding of an induction motor with its flux-density and mmf waves in their relative positions for (a) zero and (b) nonzero rotor leakage reactance.

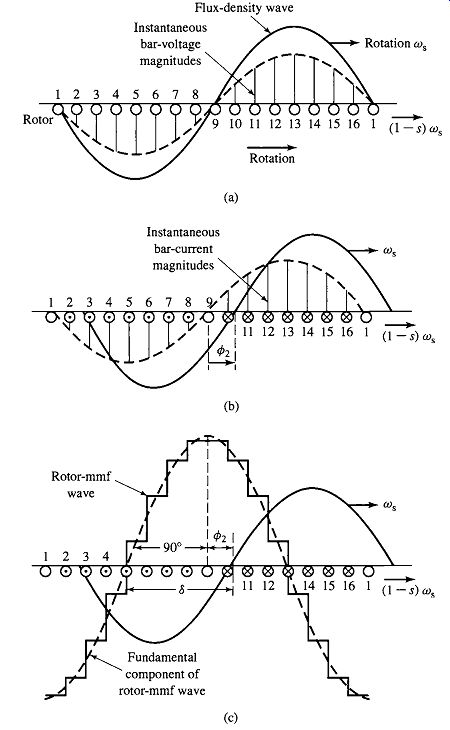
FIG. 6 Reactions of a squirrel-cage rotor in a two-pole field.

If the rotor leakage reactance is appreciable however, the phase-a current lags the induced voltage by the power-factor angle q~2 of the rotor leakage impedance. The phase-a current will not be at maximum until a correspondingly later time. The rotor mmf wave will then not be centered on phase a until the flux wave has traveled q~2 degrees farther down the gap, as shown in FIG. 5b. The angle ~r is now -(90 ° + ~b2). In general, therefore, the torque angle of an induction motor is...

(Eq. 7)

It departs from the optimum value of -90 ° by the power-factor angle of the rotor leakage impedance at slip frequency. The electromagnetic rotor torque is directed toward the right in FIG. 5, or in the direction of the rotating flux wave.

The comparable picture for a squirrel-cage rotor is given in FIG. 6. A 16-bar rotor placed in a two-pole field is shown in developed form. To simplify the drawing, only a relatively small number of rotor bars has been chosen and the number is an integral multiple of the number of poles, a choice normally avoided in order to prevent harmful harmonic effects. In FIG. 6a the sinusoidal flux-density wave induces a voltage in each bar which has an instantaneous value indicated by the solid vertical lines.

At a somewhat later instant of time, the bar currents assume the instantaneous values indicated by the solid vertical lines in FIG. 6b, the time lag corresponding to the rotor power-factor angle q~2. In this time interval, the flux-density wave has traveled in its direction of rotation with respect to the rotor through a space angle 4)2 and is then in the position shown in FIG. 6b. The corresponding rotor-mmf wave is shown by the step wave of FIG. 6c. The fundamental component is shown by the dashed sinusoid and the flux-density wave by the solid sinusoid. Study of these figures confirms the general principle that the number of rotor poles in a squirrel-cage rotor is determined by the inducing flux wave.

3. INDUCTION-MOTOR EQUIVALENT CIRCUIT

The foregoing considerations of flux and mmf waves can readily be translated to a steady-state equivalent circuit for a polyphase induction machine. In this derivation, only machines with symmetric polyphase windings excited by balanced polyphase voltages are considered. As in many other discussions of polyphase devices, it is helpful to think of three-phase machines as being Y-connected, so that currents are always line values and voltages always line-to-neutral values. In this case, we can derive the equivalent circuit for one phase, with the understanding that the voltages and currents in the remaining phases can be found simply by an appropriate phase shift of those of the phase under study (-1-120 ° in the case of a three-phase machine). First, consider conditions in the stator. The synchronously-rotating air-gap flux wave generates balanced polyphase counter emfs in the phases of the stator. The stator terminal voltage differs from the counter emf by the voltage drop in the stator leakage impedance Z1 = R1 + j X1. Thus...

(Eq. 8)

...where (Zl -Stator line-to-neutral terminal voltage...

Counter emf (line-to-neutral) generated by the resultant air-gap flux il Stator current R1 -Stator effective resistance X1 Stator leakage reactance.

The polarity of the voltages and currents are shown in the equivalent circuit of FIG. 7.

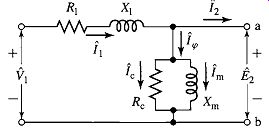
FIG. 7 Stator equivalent circuit for a polyphase induction motor.

The resultant air-gap flux is created by the combined mmf's of the stator and rotor currents. Just as in the case of a transformer, the stator current can be resolved into two components: a load component and an exciting (magnetizing) component. The load component 12 produces an mmf that corresponds to the mmf of the rotor current. The exciting component i~0 is the additional stator current required to create the resultant air-gap flux and is a function of the emf J~2. The exciting current can be resolved into a core-loss component ic in phase with /~2 and a magnetizing component Im lagging/~2 by 90 °. In the equivalent circuit, the exciting current can be accounted for by means of a shunt branch, formed by a core-loss resistance Rc and a magnetizing reactance Xm in parallel, connected across/~2, as in FIG. 7. Both Rc and Xm are usually determined at rated stator frequency and for a value of E2 close to the expected operating value; they are then assumed to remain constant for the small departures of E2 associated with normal operation of the motor.

The equivalent circuit representing stator phenomena is exactly like that used to represent the primary of a transformer. To complete our model, the effects of the rotor must be incorporated. From the point of view of the stator equivalent circuit of FIG. 7, the rotor can be represented by ...

(Eq. 9)

…corresponding to the leakage impedance of an equivalent stationary secondary. To complete the equivalent circuit, we must determine Z2 by representing the stator and rotor voltages and currents in terms of rotor quantities as referred to the stator.

As we saw in Section 2.3, from the point of view of the primary, the secondary winding of a transformer can be replaced by an equivalent secondary winding having the same number of turns as the primary winding. In a transformer where the turns ratio and the secondary parameters are known, this can be done by referring the secondary impedance to the primary by multiplying it by the square of the primary-to-secondary turns ratio. The resultant equivalent circuit is perfectly general from the point of view of primary quantities.

Similarly, in the case of a polyphase induction motor, if the rotor were to be replaced by an equivalent rotor with a polyphase winding with the same number of phases and turns as the stator but producing the same mmf and air gap flux as the actual rotor, the performance as seen from the stator terminals would be unchanged.

This concept, which we will adopt here, is especially useful in modeling squirrel-cage rotors for which the identity of the rotor "phase windings" is in no way obvious.

The rotor of an induction machine is short-circuited, and hence the impedance seen by induced voltage is simply the rotor short-circuit impedance. Consequently the relation between the slip-frequency leakage impedance Z2s of the equivalent rotor and the slip-frequency leakage impedance Zrotor of the actual rotor must be...

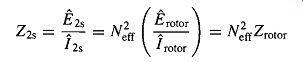
(Eq. 10)

… where Neff is the effective turns ratio between the stator winding and that of the actual rotor winding. Here the subscript 2s refers to quantities associated with the referred rotor. Thus/~2s is the voltage induced in the equivalent rotor by the resultant air-gap flux, and I2s is the corresponding induced current.

When one is concerned with the actual rotor currents and voltages, the turns ratio Neff must be known in order to convert back from equivalent-rotor quantities to those of the actual rotor. However, for the purposes of studying induction-motor performance as seen from the stator terminals, there is no need for this conversion and a representation in terms of equivalent-rotor quantities is fully adequate. Thus an equivalent circuit based upon equivalent-rotor quantities can be used to represent both coil-wound and squirrel-cage rotors.

Having taken care of the effects of the stator-to-rotor turns ratio, we next must take into account the relative motion between the stator and the rotor with the objective of replacing the actual rotor and its slip-frequency voltages and currents with an equivalent stationary rotor with stator-frequency voltages and currents. Consider first the slip-frequency leakage impedance of the referred rotor.

(Eq. 11)

...where R2 -Referred rotor resistance s X2 = Referred rotor leakage reactance at slip frequency Note that here X2 has been defined as the referred rotor leakage reactance at stator frequency fe. Since the actual rotor frequency fr Sfe, it has been converted to the slip-frequency reactance simply by multiplying by the slip s. The slip-frequency equivalent circuit of one phase of the referred rotor is shown in FIG. 8. This is the equivalent circuit of the rotor as seen in the slip-frequency rotor reference frame.

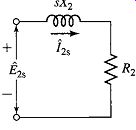
FIG. 8 Rotor equivalent circuit for a polyphase induction motor at slip frequency.

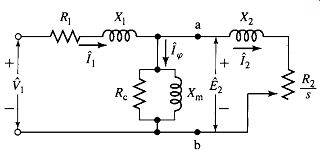
FIG. 9 Single-phase equivalent circuit for a polyphase induction motor.

We next observe that the resultant air-gap mmf wave is produced by the combined effects of the stator current il and the equivalent load current i2. Similarly, it can be expressed in terms of the stator current and the equivalent rotor current I2s. These two currents are equal in magnitude since i2s is defined as the current in an equivalent rotor with the same number of turns per phase as the stator. Because the resultant air-gap mmf wave is determined by the phasor sum of the stator current and the rotor current of either the actual or equivalent rotor, i2 and i2s must also be equal in phase (at their respective electrical frequencies) and hence we can write...

iZs = i2 (Eq. 12)

Finally, consider that the resultant flux wave induces both the slip-frequency emf induced in the referred rotor/!2s and the stator counter emf/!2. If it were not for the effect of speed, these voltages would be equal in magnitude since the referred rotor winding has the same number of turns per phase as the stator winding. However, because the relative speed of the flux wave with respect to the rotor is s times its speed with respect to the stator, the relation between these emfs is...

E2s -sE2 (Eq. 13)

We can furthermore argue that since the phase angle between each of these voltages and the resultant flux wave is 90 °, then these two voltages must also be equal in a phasor sense at their respective electrical frequencies. Hence ...

(Eq. 14)

Division of Eq. 14 by Eq. 12 and use of Eq. 11 then gives ...

Division by the slip s then gives (Eq. 15)

(Eq. 16)

We have achieved our objective. Z2 is the impedance of the equivalent stationary rotor which appears across the load terminals of the stator equivalent circuit of FIG. 7.

The final result is shown in the single-phase equivalent circuit of FIG. 9. The combined effect of shaft load and rotor resistance appears as a reflected resistance R2/s, a function of slip and therefore of the mechanical load. The current in the reflected rotor impedance equals the load component I2 of stator current; the voltage across this impedance equals the stator voltage/~2. Note that when rotor currents and voltages are reflected into the stator, their frequency is also changed to stator frequency. All rotor electrical phenomena, when viewed from the stator, become stator-frequency phenomena, because the stator winding simply sees mmf and flux waves traveling at synchronous speed.

4. ANALYSIS OF THE EQUIVALENT CIRCUIT

The single-phase equivalent circuit of FIG. 9 can be used to determine a wide variety of steady-state performance characteristics of polyphase induction machines.

These include variations of current, speed, and losses as the load-torque requirements change, as well as the starting torque, and the maximum torque.

The equivalent circuit shows that the total power P-gap transferred across the air gap from the stator is...

(Eq. 17)

...where nph is the number of stator phases.

The total rotor I ZR loss,/°rotor, can be calculated from the I ZR loss in the equivalent rotor as (Eq. 18)...

Since I2s = 12, we can write Eq. 18 as...

(Eq. 19)

The electromagnetic power Pmech developed by the motor can now be determined by subtracting the rotor power dissipation of Eq. 19 from the air-gap power of Eq. 17.

(Eq. 20)

...or equivalently...

(Eq. 21)

Comparing Eq. 17 with Eq. 21 gives...

(Eq. 22)

(Eq. 23)

We see then that, of the total power delivered across the air gap to the rotor, the fraction 1 s is converted to mechanical power and the fraction s is dissipated as 12 R loss in the rotor conductors. From this it is evident that an induction motor operating at high slip is an inefficient device. When power aspects are to be emphasized, the equivalent circuit can be redrawn in the manner of FIG. 10. The electromechanical power per stator phase is equal to the power delivered to the resistance R2(1 s)/s.

The electromechanical Tmech corresponding to the power emech can be obtained by recalling that mechanical power equals torque times angular velocity. Thus,...

(Eq. 24)

For Pmech in watts and cos in rad/sec, Tmech will be in newton-meters.

Use of Eqs. 21 and 22 leads to

(Eq. 25)

… with the synchronous mechanical angular velocity COs being given by

…(Eq. 26)

The mechanical torque Tmech and power Pmech are not the output values available at the shaft because friction, windage, and stray-load losses remain to be accounted for. It is obviously correct to subtract friction, windage, and other rotational losses from Tmech or Pmech and it is generally assumed that stray load effects can be subtracted in the same manner. The remainder is available as output power from the shaft for useful work. Thus and Pshaftemecherot (Eq. 27)...

eshaft Tshaft~--TmechTrot (Eq. 28)

... where erot and Tro t are the power and torque associated with the friction, windage, and remaining rotational losses.

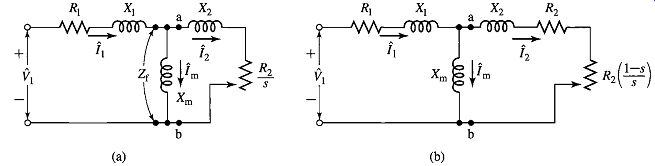
FIG. 11 Equivalent circuits with the core-loss resistance Rc neglected corresponding to (a) FIG. 9 and (b) FIG. 10.

Analysis of the transformer equivalent circuit is often simplified by either neglecting the magnetizing branch entirely or adopting the approximation of moving it out directly to the primary terminals. Such approximations are not used in the case of induction machines under normal running conditions because the presence of the air gap results in a relatively lower magnetizing impedance and correspondingly a relatively higher exciting current m 30 to 50 percent of full-load current m and because the leakage reactances are also higher. Some simplification of the induction-machine equivalent circuit results if the core-loss resistance Rc is omitted and the associated core-loss effect is deducted from Tmech or Pmech at the same time that rotational losses and stray load effects are subtracted. The equivalent circuit then becomes that of FIG. 11a or b, and the error introduced is often relatively insignificant. Such a procedure also has an advantage during motor testing, for then the no-load core loss need not be separated from friction and windage. These last circuits are used in subsequent discussions.

5. TORQUE AND POWER BY USE OF THEVENIN'S THEOREM

When torque and power relations are to be emphasized, considerable simplification results from application of Thevenin's network theorem to the induction-motor equivalent circuit. In its general form, Thevenin's theorem permits the replacement of any network of linear circuit elements and complex voltage sources, such as viewed from two terminals a and b (FIG. 12a), by a single complex voltage source Veq in series with a single impedance Zeq (FIG. 12b). The Thevenin-equivalent voltage l?eq is that appearing across terminals a and b of the original network when these terminals are open-circuited; the Thevenin-equivalent impedance Zeq is that viewed from the same terminals when all voltage sources within the network are set equal to zero.

For application to the induction-motor equivalent circuit, points a and b are taken as those so designated in FIG. 11 a and b. The equivalent circuit then assumes the forms given in FIG. 13 where Thevenin's theorem has been used to transform the network to the left of points a and b into an equivalent voltage source f'],eq in series with an equivalent impedance Z],eq = R l,eq n tj X l,eq.

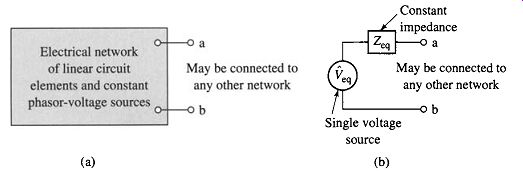
FIG. 12 (a) General linear network and (b)its equivalent at terminals ab by Thevenin's theorem.

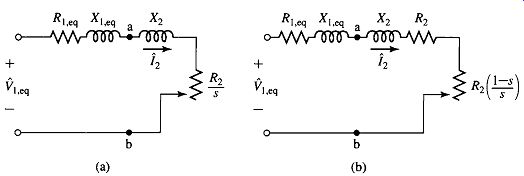
FIG. 13 Induction-motor equivalent circuits simplified by Thevenin's theorem.

According to Thevenin's theorem, the equivalent source voltage 01,eq is the voltage that would appear across terminals a and b of FIG. 11 with the rotor circuits removed. The result is a simple voltage divider and thus

… (Eq. 29)

For most induction motors, negligible error results from neglecting the stator resistance in Eq. 29. The Thevenin-equivalent stator impedance Zl,eq is the impedance between terminals a and b of FIG. 11 viewed toward the source with the source voltage set equal to zero (or equivalently replaced by a short circuit) and therefore is

… (Eq. 31)

Note that the core-loss resistance Rc has been neglected in the derivation of Eqs. 29 through 31. Although this is a very commonly used approximation, its effect can be readily incorporated in the derivations presented here by replacing the magnetizing reactance j Xm by the magnetizing impedance Zm, equal to the parallel combination of the core-loss resistance Rc and the magnetizing reactance j Xm.

From the Thevenin-equivalent circuit (FIG. 13)

(Eq. 32)

… (Eq. 25)

…(Eq. 33)

…. where COs is the synchronous mechanical angular velocity as given by Eq. 26. The general shape of the torque-speed or torque-slip curve with the motor connected to a constant-voltage, constant-frequency source is shown in Figs. 6.14 and 6.15.

In normal motor operation, the rotor revolves in the direction of rotation of the magnetic field produced by the stator currents, the speed is between zero and synchronous speed, and the corresponding slip is between 1.0 and 0 (labeled "Motor region" in FIG. 14). Motor starting conditions are those of s -1.0.

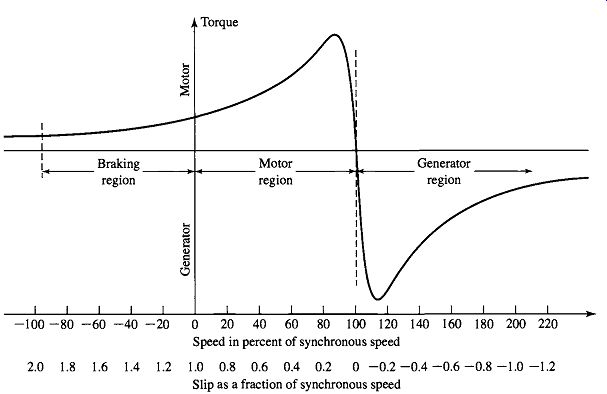
FIG. 14 Induction-machine torque-slip curve showing braking, motor, and generator regions.

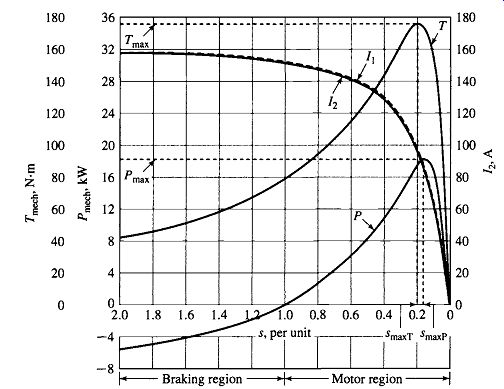
FIG. 15 Computed torque, power, and current curves for the 7.5-kW motor in Examples 2 and 3.

To obtain operation in the region of s greater than 1 (corresponding to a negative motor speed), the motor must be driven backward, against the direction of rotation of its magnetic field, by a source of mechanical power capable of counteracting the electromechanical torque Tmech. The chief practical usefulness of this region is in bringing motors to a quick stop by a method called plugging. By interchanging two stator leads in a three-phase motor, the phase sequence, and hence the direction of rotation of the magnetic field, is reversed suddenly and what was a small slip before the phase reversal becomes a slip close to 2.0 following the reversal; the motor comes to a stop under the influence of torque Tmech and is disconnected from the line before it can start in the other direction. Accordingly, the region from s = 1.0 to s -2.0 is labeled "Braking region" in FIG. 14.

The induction machine will operate as a generator if its stator terminals are connected to a polyphase voltage source and its rotor is driven above synchronous speed (resulting in a negative slip) by a prime mover, as shown in FIG. 14. The source fixes the synchronous speed and supplies the reactive power input required to excite the air-gap magnetic field. One such application is that of an induction generator connected to a power system and driven by a wind turbine.

An expression for the maximum electromechanical torque, or breakdown torque, ...

Tmax, indicated in FIG. 15, can be obtained readily from circuit considerations. As can be seen from Eq. 25, the electromechanical torque is a maximum when the power delivered to R2/s in FIG. 13a is a maximum. It can be shown that this power will be greatest when the impedance of R2/s equals the magnitude of the impedance Rl, eq -+j (Xl,eq + X2) between it and the constant equivalent voltage (Zl,eq. Thus, maximum electromechanical torque will occur at a value of slip (Eq. 34)...

(Eq. 35)

...and the corresponding torque is, from Eq. 33,...

(Eq. 36)

...where COs is the synchronous mechanical angular velocity as given by Eq. 26.

Under the conditions of constant-frequency operation, a typical conventional induction motor with a squirrel-cage rotor is substantially a constant-speed motor having about 10 percent or less drop in speed from no load to full load. In the case of a wound-rotor induction motor, speed variation can be obtained by inserting external resistance in the rotor circuit; the influence of increased rotor resistance on the torque-speed characteristic is shown by the dashed curves in FIG. 16. For such a motor, significant speed variations can be achieved as the rotor resistance is varied. Similarly, the zero-speed torque variations seen in FIG. 16 illustrate how the starting torque of a wound-rotor induction motor can be varied by varying the rotor resistance.

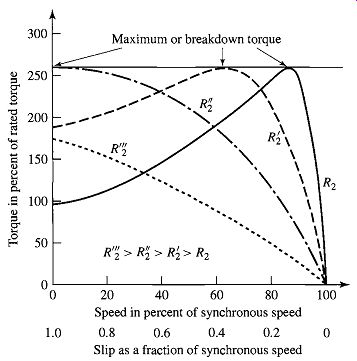
FIG. 16 Induction-motor torque-slip curves showing effect of changing rotor-circuit resistance.

Notice from Eqs. 35 and 36 that the slip at maximum torque is directly proportional to rotor resistance R2 but the value of the maximum torque is independent of R2. When R2 is increased by inserting external resistance in the rotor of a wound-rotor motor, the maximum electromechanical torque is unaffected but the speed at which it occurs can be directly controlled. This result can also be seen by observing that the electromechanical torque expression of Eq. 33 is a function of the ratio R2/s. Thus, the torque is unchanged as long as the ratio R2/s remains constant.

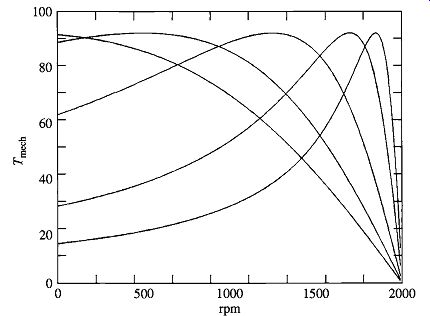
FIG. 17 Electromechanical torque vs. speed for the wound-rotor induction motor of Example 4 for various values of the rotor resistance R2.

In applying the induction-motor equivalent circuit, the idealizations on which it is based should be kept in mind. This is particularly necessary when investigations are carried out over a wide speed range, such as is the case in investigations of motor starting. Saturation under the heavy inrush currents associated with starting conditions has a significant effect on the motor reactances. Moreover, the rotor currents are at slip frequency, which varies from stator frequency at zero speed to a low value at full-load speed. The current distribution in the rotor bars of squirrel-cage motors may vary significantly with frequency, giving rise to significant variations in rotor resistance. In fact, as discussed in Sections 7.2 and 7.3, motor designers can tailor the shape of the rotor bars in squirrel-cage motors to obtain various speed-torque characteristics. Errors from these causes can be kept to a minimum by using equivalent-circuit parameters corresponding as closely as possible to those of the proposed operating conditions.

6. PARAMETER DETERMINATION FROM NO-LOAD AND BLOCKED-ROTOR TESTS

The equivalent-circuit parameters needed for computing the performance of a polyphase induction motor under load can be obtained from the results of a no-load test, a blocked-rotor test, and measurements of the dc resistances of the stator windings.

Stray-load losses, which must be taken into account when accurate values of efficiency are to be calculated, can also be measured by tests which do not require loading the motor. The stray-load-loss tests are not described here, however.

[See, for instance, R. E Horrell and W. E. Wood, "A Method of Determining Induction Motor Speed-Torque-Current Curves from Reduced Voltage Tests," Trans. AIEE, 73(3):670-674 (1954). 2 For information concerning test methods, see IEEE Std. 112-1996, "Test Procedures for Polyphase Induction Motors and Generators," Institute of Electrical and Electronics Engineers, Inc., 345 East 47th Street, New York, New York, 10017. ]

Like the open-circuit test on a transformer, the no-load test on an induction motor gives information with respect to exciting current and no-load losses. This test is ordinarily performed at rated frequency and with balanced polyphase voltages applied to the stator terminals. Readings are taken at rated voltage, after the motor has been running long enough for the bearings to be properly lubricated. We will assume that the no-load test is made with the motor operating at its rated electrical frequency fr and that the following measurements are available from the no-load test: Vl,nl The line-to-neutral voltage [V] II,nl -The line current [V] Pnl -The total polyphase electrical input power [W] In polyphase machines it is most common to measure line-to-line voltage, and thus the phase-to-neutral voltage must be then calculated (dividing by ~/3 in the case of a three-phase machine). At no load, the rotor current is only the very small value needed to produce sufficient torque to overcome the friction and windage losses associated with rotation.

The no-load rotor IZR loss is, therefore, negligibly small. Unlike the continuous magnetic core in a transformer, the magnetizing path in an induction motor includes an air gap which significantly increases the required exciting current. Thus, in contrast to the case of a transformer, whose no-load primary I^2 R loss is negligible, the no-load stator I 2 R loss of an induction motor may be appreciable because of this larger exciting current.

Neglecting rotor I^2 R losses, the rotational loss Prot for normal running conditions can be found by subtracting the stator IZR losses from the no-load input power...

(Eq. 37)

The total rotational loss at rated voltage and frequency under load usually is considered to be constant and equal to its no-load value. Note that the stator resistance R1 varies with stator-winding temperature. Hence, when applying Eq. 37, care should be taken to use the value corresponding to the temperature of the no-load test.

Note that the derivations presented here ignore the core-loss and the associated core-loss resistance and assign all the no-load losses to friction and windage. Various tests can be performed to separate the friction and windage losses from the core losses.

For example, if the motor is not energized, an external drive motor can be used to drive the rotor to the no-load speed and the rotational loss will be equal to the required drive-motor output power.

Alternatively, if the motor is operated at no load and rated speed and if it is then suddenly disconnected from the supply, the decay in motor speed will be determined by the rotational loss as...

(Eq. 38)

Hence, if the rotor inertia J is known, the rotational loss at any speed (- Om can be determined from the resultant speed decay as...

(Eq. 39)

dt Thus, the rotational losses at rated speed can be determined by evaluating Eq. 39 as the motor is first shut off when it is operating at rated speed.

If the no-load rotational losses are determined in this fashion, the core loss can be determined as ...

(Eq. 40)

Here Pcore is the total no-load core loss corresponding to the voltage of the no-load test (typically rated voltage).

Under no-load conditions, the stator current is relatively low and, to a first approximation, one can neglect the corresponding voltage drop across the stator resistance and leakage reactance. Under this approximation, the voltage across the core-loss resistance will be equal to the no-load line-to-neutral voltage and the core-loss resistance can be determined as...

(Eq. 41)

Provided that the machine is operated close to rated speed and rated voltage, the refinement associated with separating out the core loss and specifically incorporating it in the form of a core-loss resistance in the equivalent circuit will not make a significant difference in the results of an analysis. Hence, it is common to ignore the core-loss resistance and to simply include the core losses with the rotational losses. For the purposes of analytical simplicity, this approach will be followed in the remainder of the text. However, if necessary, the reader should find it relatively straight forward to modify the remaining derivations to appropriately include the core-loss resistance.

Because the slip at no load, Snl, is very small, the reflected rotor resistance R2/Snl is very large. The parallel combination of rotor and magnetizing branches then becomes j Xm shunted by the rotor leakage reactance X2 in series with a very high resistance, and the reactance of this parallel combination therefore very nearly equals Xm. Consequently the apparent reactance Xnl measured at the stator terminals at no load very nearly equals…

...which is the self-reactance X1~ of the stator; i.e.,

(Eq. 42)

The self-reactance of the stator can therefore be determined from the no-load measurements. The reactive power at no load Qnl can be determined as….

(Eq. 44)

is the total apparent power input at no load.

The no-load reactance Xnl can then be calculated from:

(Eq. 45)

Usually the no-load power factor is small (i.e., anl >> enl) SO that the no-load reactance very nearly equals the no-load impedance.

(Eq. 46)

6.2 Blocked-Rotor Test

Like the short-circuit test on a transformer, the blocked-rotor test on an induction motor gives information with respect to the leakage impedances. The rotor is blocked so that it cannot rotate (hence the slip is equal to unity), and balanced polyphase voltages are applied to the stator terminals. We will assume that the following measurements are available from the blocked-rotor test:

The line-to-neutral voltage [V]

II,bl -The line current [V]

Pbl -The total polyphase electrical input power [W]

fbl = The frequency of the blocked-rotor test [Hz]

In some cases, the blocked-rotor torque also is measured.

The equivalent circuit for blocked-rotor conditions is identical to that of a short-circuited transformer. An induction motor is more complicated than a transformer, however, because its leakage impedance may be affected by magnetic saturation of the leakage-flux paths and by rotor frequency. The blocked-rotor impedance may also be affected by rotor position, although this effect generally is small with squirrel-cage rotors.

The guiding principle is that the blocked-rotor test should be performed under conditions for which the current and rotor frequency are approximately the same as those in the machine at the operating condition for which the performance is later to be calculated. For example, if one is interested in the characteristics at slips near unity, as in starting, the blocked-rotor test should be taken at normal frequency and with currents near the values encountered in starting. If, however, one is interested in normal running characteristics, the blocked-rotor test should be taken at a reduced voltage which results in approximately rated current; the frequency also should be reduced, since the values of rotor effective resistance and leakage inductance at the low rotor frequencies corresponding to small slips may differ appreciably from their values at normal frequency, particularly with double-cage or deep-bar rotors, as discussed in Section 7.2.

IEEE Standard 112 suggests a blocked-rotor test frequency of 25 percent of rated frequency. The total leakage reactance at normal frequency can be obtained from this test value by considering the reactance to be proportional to frequency. The effects of frequency often are negligible for normal motors of less than 25-hp rating, and the blocked impedance can then be measured directly at normal frequency. The importance of maintaining test currents near their rated value stems from the fact that these leakage reactances are significantly affected by saturation.

Based upon blocked-rotor measurements, the blocked-rotor reactance can be found from the blocked-rotor reactive power

(Eq. 47)

...where ...

(Eq. 48)

is the total blocked-rotor apparent power. The blocked-rotor reactance, corrected to rated frequency, can then be calculated as

(Eq. 49)

The blocked-rotor resistance can be calculated from the blocked-rotor input power as...

(Eq. 50)

Once these parameters have been determined, the equivalent circuit parameters can be determined. Under blocked-rotor conditions, an expression for the stator input impedance can be obtained from examination of FIG. 11 a (with s = 1) as

… in parallel with […]

Here we have assumed that the reactances are at their rated-frequency values. Making appropriate approximations (e.g., assuming R2 << Xm), Eq. 51 can be reduced to:

(Eq. 52)

Thus the apparent resistance under blocked-rotor conditions is given by:

(Eq. 53)

and the apparent rated-frequency blocked-rotor reactance by:

(Eq. 54)

From Eqs. 54 and 53, the rotor leakage reactance X2 and resistance R2 can be found as ( Xm ) (Eq. 55)

(Eq. 56)

Table 1 Empirical distribution of leakage reactances in induction motors.

In order to achieve maximum accuracy as with the no-load test, if possible the value of the stator resistance R1 used in Eq. 56 should be corrected to the value corresponding to the temperature of the blocked-rotor test.

Substituting for Xm from Eq. 42 into Eq. 55 gives…

(Eq. 57)

Equation 57 expresses the rotor leakage reactance X2 in terms of the measured quantities Xnl and Xbl and the unknown stator leakage reactance X1. It is not possible to make an additional measurement from which X1 and X2 can be determined uniquely.

Fortunately, the performance of the motor is affected relatively little by the way in which the total leakage reactance is distributed between the stator and rotor. IEEE Standard 112 recommends the empirical distribution shown in Table 1. If the motor class is unknown, it is common to assume that X1 and X2 are equal.

Once the fractional relationship between X1 and X2 has been determined, it can be substituted into Eq. 57 and X2 (and hence X1) can be found in terms of Xnl and Xbl by solving the resultant quadratic equation.

The magnetizing reactance Xm can then be determined from Eq. 42.

(Eq. 58)

Finally, using the known stator resistance and the values of Xm and X2 which are now known, the rotor resistance R2 can now be determined from Eq. 56.

7. EFFECTS OF ROTOR RESISTANCE; WOUND AND DOUBLESQUIRREL-CAGE ROTORS

A basic limitation of induction motors with constant rotor resistance is that the rotor design has to be a compromise. High efficiency under normal running conditions requires a low rotor resistance; but a low rotor resistance results in a low starting torque and high starting current at a low starting power factor.

7.1 Wound-Rotor Motors

The use of a wound rotor is one effective way of avoiding the need for compromise. The terminals of the rotor winding are connected to slip rings in contact with brushes. For starting, resistors may be connected in series with the rotor windings, the result being increased starting torque and reduced starting current at an improved power factor.

The general nature of the effects on the torque-speed characteristics caused by varying rotor resistance is shown in FIG. 16. By use of the appropriate value of rotor resistance, the maximum torque can be made to occur at standstill if high starting torque is needed. As the rotor speeds up, the external resistances can be decreased, making maximum torque available throughout the accelerating range. Since most of the rotor I^2R loss is dissipated in the external resistors, the rotor temperature rise during starting is lower than it would be if the resistance were incorporated in the rotor winding. For normal running, the rotor winding can be short-circuited directly at the brushes. The rotor winding is designed to have low resistance so that running efficiency is high and full-load slip is low. Besides their use when starting requirements are severe, wound-rotor induction motors can be used for adjustable-speed drives.

Their chief disadvantage is greater cost and complexity than squirrel-cage motors.

The principal effects of varying rotor resistance on the starting and running characteristics of induction motors can be shown quantitatively by the following example.

EXAMPLE 7

A three-phase, 460-V, 60-Hz, four-pole, 500-hp wound-rotor induction motor, with its slip tings short-circuited, has the following properties:

Full-load slip = 1.5 percent

Rotor IZR at full-load torque = 5.69 kW

Slip at maximum torque = 6 percent

Rotor current at maximum torque = 2.8212,fl, where I2,el is the full-load rotor current

Torque at 20 percent slip = 1.20Tfl, where Tel is the full-load torque Rotor current at 20 percent slip -3.95 I2,fl If the rotor-circuit resistance is increased to 5 R rotor by connecting noninductive resistances in series with each rotor slip ring, determine (a) the slip at which the motor will develop the same full-load torque, (b) total rotor-circuit IZR loss at full-load torque, (c) horsepower output at full-load torque, (d) slip at maximum torque, (e) rotor current at maximum torque, (f) starting torque, and (g) rotor current at starting. Express the torques and rotor currents in per unit based on the full-load torque values.

Solution

The solution involves recognition of the fact that the effects of changes in the rotor resistance are seen from the stator in terms of changes in the referred resistance R2/s. Examination of the equivalent circuit shows that, for specified applied voltage and frequency, everything concerning the stator performance is fixed by the value of R2/s, the other impedance elements being constant. For example, if R2 is doubled and s is simultaneously doubled, there will be no indication from the stator that anything has changed. The stator current and power factor, the power delivered to the air gap, and the torque will be unchanged as long as the ratio Rz/S remains constant.

Added physical significance can be given to the argument by examining the effects of simultaneously doubling R2 and s from the viewpoint of the rotor. An observer on the rotor would see the resultant air-gap flux wave traveling past at twice the original slip speed, generating twice the original rotor voltage at twice the original slip frequency. The rotor reactance therefore is doubled, and since the original premise is that the rotor resistance also is doubled, the rotor impedance is doubled while the rotor power factor is unchanged. Since rotor voltage and impedance are both doubled, the effective value of the rotor current remains the same; only its frequency is changed. The air gap still has the same synchronously rotating flux and mmf waves with the same torque angle. An observer on the rotor would then agree with a counterpart on the stator that the torque is unchanged.

An observer on the rotor, however, would be aware of two changes not apparent in the stator: (1) the rotor I 2 R loss will doubled, and (2) the rotor is turning more slowly and therefore developing less mechanical power with the same torque. In other words, more of the power absorbed from the stator goes into 12 R heat in the rotor, and less is available for mechanical power.

The preceding thought processes can be readily applied to the solution of this example.

a. If the rotor resistance is increased five times, the slip must increase five times for the same value of Rz/s and therefore for the same torque. But the original slip at full load is 0.015.

The new slip at full-load torque therefore is 5(0.015) = 0.075.

b. The effective value of the rotor current is the same as its full-load value before addition of the series resistance, and therefore the rotor Rz/s loss is five times the full-load value of 5.69 kW, or Rotor 12R = 5 × 5.69 = 28.4 kW

c. The increased slip has caused the per-unit speed at full-load torque to drop from 1 s = 0.985 down to 1 s = 0.925. Since the ratio R2/s is unchanged, the torque is the same and hence the power output has dropped proportionally, or 0.925 Pmech = (500) = 470 hp

Because the air-gap power is unchanged, the decrease in electromechanical mechanical shaft power must be accompanied by a corresponding increase in rotor I ZR loss.

d. If rotor resistance is increased five times, the slip at maximum torque simply increases five times. But the original slip at maximum torque is 0.060. The new slip at maximum torque with the added rotor resistance therefore is

SmaxT -5(0.060) = 0.30

e. The effective value of the rotor current at maximum torque is independent of rotor resistance; only its frequency is changed when rotor resistance is varied. Therefore, 12 .... T = 2.8212,fl

f. With the rotor resistance increased five times, the starting torque will be the same as the original running torque at a slip of 0.20 and therefore equals the running torque without the series resistors, namely, Tstart = 1.20Tel

g. The rotor current at starting with the added rotor resistances will be the same as the rotor current when running at a slip of 0.20 with the slip rings short-circuited, namely, I2,~t,, = 3.9512,fl

7.2 Deep-Bar and Double-Squirrel-Cage Rotors

An ingenious and simple way of obtaining a rotor resistance which will automatically vary with speed makes use of the fact that at standstill the rotor frequency equals the stator frequency; as the motor accelerates, the rotor frequency decreases to a very low value, perhaps 2 or 3 Hz at full load in a 60-Hz motor. With suitable shapes and arrangements for rotor bars, squirrel-cage rotors can be designed so that their effective resistance at 60 Hz is several times their resistance at 2 or 3 Hz. The various schemes all make use of the inductive effect of the slot-leakage flux on the current distribution in the rotor bars. This phenomenon is similar to the skin and proximity effect in any system of conductors carrying alternating current.

Consider first a squirrel-cage rotor having deep, narrow bars like that shown in cross section in FIG. 18. The general character of the slot-leakage field produced by the current in the bar within this slot is shown in the figure. If the rotor iron had infinite permeability, all the leakage-flux lines would close in paths below the slot, as shown. Now imagine the bar to consist of an infinite number of layers of differential depth; one at the bottom and one at the top are indicated crosshatched in FIG. 18. The leakage inductance of the bottom layer is greater than that of the top layer because the bottom layer is linked by more leakage flux. Because all the layers are electrically in parallel, under ac conditions, the current in the low-reactance upper layers will be greater than that in the high-reactance lower layers. As a result, the current will be forced toward the top of the slot, and the phase of current in the upper layers will lead that of the current in the lower ones.

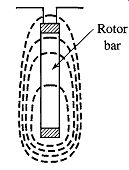
FIG. 18 Deep rotor bar and slot-leakage flux.

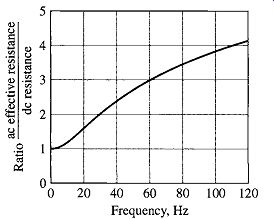
FIG. 19 Skin effect in a copper rotor bar 2.5 cm deep.

This nonuniform current distribution results in an increase in the effective bar resistance and a smaller decrease in the effective leakage inductance of the bar. Since the distortion in current distribution depends on an inductive effect, the effective resistance is a function of the frequency. It is also a function of the depth of the bar and of the permeability and resistivity of the bar material. FIG. 19 shows a curve of the ratio of effective ac resistance to dc resistance as a function of frequency computed for a copper bar 2.5 cm deep. A squirrel-cage rotor with deep bars can be readily designed to have an effective resistance at stator frequency (corresponding to rotor standstill conditions) several times greater than its dc resistance. As the motor accelerates, the rotor frequency decreases and therefore the effective rotor resistance decreases, approaching its dc value at small slips.

FIG. 20 Double-squirrel-cage rotor bars and slot-leakage flux.

An alternative way of attaining similar results is the double-cage arrangement shown in FIG. 20. In this case, the squirrel-cage winding consists of two layers of bars short-circuited by end tings. The upper bars are of smaller cross-sectional area than the lower bars and consequently have higher resistance. The general nature of the slot-leakage field is shown in FIG. 20, from which it can be seen that the inductance of the lower bars is greater than that of the upper ones because of the flux crossing the slot between the two layers. The difference in inductance can be made quite large by properly proportioning the constriction in the slot between the two bars. At standstill, when rotor frequency equals stator frequency, there is relatively little current in the lower bars because of their high reactance; the effective resistance of the rotor at standstill is then approximately equal to that of the high-resistance upper layer. At the low rotor frequencies corresponding to small slips, however, reactance effects become negligible, and the rotor resistance then approaches that of the two layers in parallel.

Note that since the effective resistance and leakage inductance of double-cage and deep-bar rotors vary with frequency, the parameters R2 and X2, representing the referred effects of rotor resistance and leakage inductance as viewed from the stator, vary with rotor speed and are not constant. Strictly speaking, a more complicated form of equivalent circuit, with multiple parallel branches, is required in order to represent these cases.

Under steady-state conditions, the simple equivalent circuit derived in Section 3 can still be used to represent induction machines in these cases. However R2 and X2 must be varied with slip. All the basic relations still apply to the motor if the values of R2 and X2 are properly adjusted with changes in slip. For example, in computing the starting performance, R2 and X2 should be taken as their effective values at stator frequency, while in computing the running performance at small slips, R2 should be taken as its effective value at a low frequency, and X2 should be taken as the stator-frequency value of the reactance corresponding to a low-frequency effective value of the rotor leakage inductance. Over the normal running range of slips, the rotor resistance and leakage inductance usually can be considered constant at substantially their dc values.

7.3 Motor-Application Considerations

By use of double-cage and deep-bar rotors, squirrel-cage motors can be designed to have the good starting characteristics resulting from high rotor resistance and, at the same time, the good running characteristics resulting from low rotor resistance. The design is necessarily somewhat of a compromise, however, and such motors lack the flexibility of a wound-rotor machine with external rotor resistance. As a result, wound-rotor motors were commonly preferred when starting requirements were severe. However, as discussed in Section 11.3, when combined with power-electronics, squirrel-cage motors can achieve all the flexibility of wound-rotor motors, and hence wound-rotor motors are becoming increasingly less common even in these cases.

To meet the usual needs of industry, integral-horsepower, three-phase, squirrel-cage motors are available from manufacturers' stock in a range of standard ratings up to 200 hp at various standard frequencies, voltages, and speeds. (Larger motors are generally regarded as special-purpose rather than general-purpose motors.) Several standard designs are available to meet various starting and running requirements.

Representative torque-speed characteristics of the four most common designs are shown in FIG. 21. These curves are fairly typical of 1800 r/min (synchronous-speed) motors in ratings from 7.5 to 200 hp although it should be understood that individual motors may differ appreciably from these average curves.

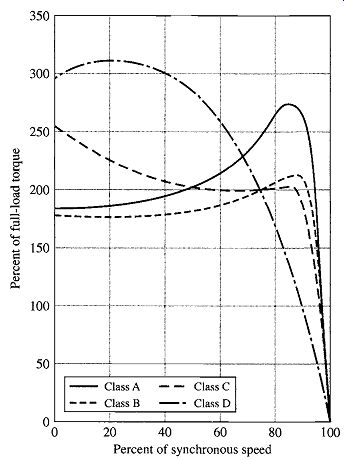
FIG. 21 Typical torque-speed curves for 1800-r/min general-purpose induction motors.

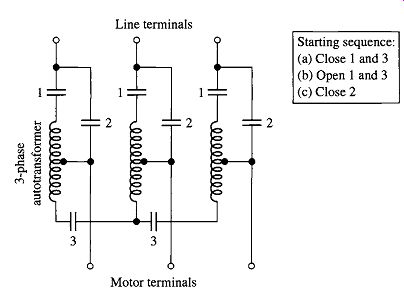
FIG. 22 Connections of a one-step starting autotransformer.

Briefly, the characteristic features of these designs are as follows.

Design Class A: Normal Starting Torque, Normal Starting Current, Low Slip

This design usually has a low-resistance, single-cage rotor. It emphasizes good running performance at the expense of starting. The full-load slip is low and the full-load efficiency is high. The maximum torque usually is well over 200 percent of full-load torque and occurs at a small slip (less than 20 percent). The starting torque at full voltage varies from about 200 percent of full-load torque in small motors to about 100 percent in large motors. The high starting current (500 to 800 percent of full-load current when started at rated voltage) is the principal disadvantage of this design.

In sizes below about 7.5 hp these starting currents usually are within the limits on inrush current which the distribution system supplying the motor can withstand, and across-the-line starting at full voltage then can be used. Otherwise, reduced-voltage starting must be used. Reduced-voltage starting results in a decrease in starting torque because the starting torque is proportional to the square of the voltage applied to the motor terminals. The reduced voltage for starting is usually obtained from an autotransformer, called a starting compensator, which may be manually operated or automatically operated by relays which cause full voltage to be applied after the motor is up to speed. A circuit diagram of one type of compensator is shown in FIG. 22. If a smoother start is necessary, series resistance or reactance in the stator may be used.

The class A motor is the basic standard design in sizes below about 7.5 and above about 200 hp. It is also used in intermediate ratings where design considerations may make it difficult to meet the starting-current limitations of the class-B design. Its field of application is about the same as that of the class-B design described next.

Design Class B: Normal Starting Torque, Low Starting Current, Low Slip

This design has approximately the same starting torque as the class-A design with but 75 percent of the starting current. Full-voltage starting, therefore, may be used with larger sizes than with class A. The starting current is reduced by designing for relatively high leakage reactance, and the starting torque is maintained by use of a double-cage or deep-bar rotor. The full-load slip and efficiency are good, about the same as for the class A design. However, the use of high reactance slightly decreases the power factor and decidedly lowers the maximum torque (usually only slightly over 200 percent of full-load torque being obtainable). This design is the most common in the 7.5 to 200-hp range of sizes. It is used for substantially constant-speed drives where starting-torque requirements are not severe, such as in driving fans, blowers, pumps, and machine tools.

Design Class C: High Starting Torque, Low Starting Current

This design uses a double-cage rotor with higher rotor resistance than the class-B design. The result is higher starting torque with low starting current but somewhat lower running efficiency and higher slip than the class-A and class-B designs. Typical applications are in driving compressors and conveyers.

Design Class D: High Starting Torque, High Slip

This design usually has a single-cage, high-resistance rotor (frequently brass bars). It produces very high starting torque at low starting current, high maximum torque at 50 to 100 percent slip, but runs at a high slip at full load (7 to 11 percent) and consequently has low running efficiency.

Its principal uses are for driving intermittent loads involving high accelerating duty and for driving high-impact loads such as punch presses and shears. When driving high-impact loads, the motor is generally aided by a flywheel which helps supply the impact and reduces the pulsations in power drawn from the supply system. A motor whose speed falls appreciably with an increase in torque is required so that the flywheel can slow down and deliver some of its kinetic energy to the impact.

8. SUMMARY

In a polyphase induction motor, slip-frequency currents are induced in the rotor windings as the rotor slips past the synchronously-rotating stator flux wave. These rotor currents, in turn, produce a flux wave which rotates in synchronism with the stator flux wave; torque is produced by the interaction of these two flux waves. For increased load on the motor, the rotor speed decreases, resulting in larger slip, increased induced rotor currents, and greater torque.

Examination of the flux-mmf interactions in a polyphase induction motor shows that, electrically, the machine is a form of transformer. The synchronously-rotating air-gap flux wave in the induction machine is the counterpart of the mutual core flux in the transformer. The rotating field induces emf's of stator frequency in the stator windings and of slip frequency in the rotor windings (for all rotor speeds other than synchronous speed). Thus, the induction machine transforms voltages and at the same time changes frequency. When viewed from the stator, all rotor electrical and magnetic phenomena are transformed to stator frequency. The rotor mmf reacts on the stator windings in the same manner as the mmf of the secondary current in a transformer reacts on the primary. Pursuit of this line of reasoning leads to a single-phase equivalent circuit for polyphase induction machines which closely resemble that of a transformer.

For applications requiting a substantially constant speed without excessively severe starting conditions, the squirrel-cage motor usually is unrivaled because of its ruggedness, simplicity, and relatively low cost. Its only disadvantage is its relatively low power factor (about 0.85 to 0.90 at full load for four-pole, 60-Hz motors and considerably lower at light loads and for lower-speed motors). The low power factor is a consequence of the fact that all the excitation must be supplied by lagging reactive power taken from the ac source.

One of the salient facts affecting induction-motor applications is that the slip at which maximum torque occurs can be controlled by varying the rotor resistance.

A high rotor resistance gives optimum starting conditions but poor running performance. A low rotor resistance, however, may result in unsatisfactory starting conditions. However, the design of a squirrel-cage motor is, therefore, quite likely to be a compromise.

Marked improvement in the starting performance with relatively little sacrifice in running performance can be built into a squirrel-cage motor by using a deep-bar or double-cage rotor whose effective resistance increases with slip. A wound-rotor motor can be used for very severe starting conditions or when speed control by rotor resistance is required. Variable-frequency solid-state motor drives lend considerable flexibility to the application of induction motors in variable-speed applications. These issues are discussed in Section 11.

9. QUIZ

1. The nameplate on a 460-V, 50-hp, 60-Hz, four-pole induction motor indicates that its speed at rated load is 1755 r/min. Assume the motor to be operating at rated load.

a. What is the slip of the rotor?

b. What is the frequency of the rotor currents?

c. What is the angular velocity of the stator-produced air-gap flux wave with respect to the stator? With respect to the rotor?

d. What is the angular velocity of the rotor-produced air-gap flux wave with respect to the stator? With respect to the rotor?

2. Stray leakage fields will induce rotor-frequency voltages in a pickup coil mounted along the shaft of an induction motor. Measurement of the frequency of these induced voltages can be used to determine the rotor speed.

a. What is the rotor speed in r/min of a 50-Hz, six-pole induction motor if the frequency of the induced voltage is 0.89 Hz?

b. Calculate the frequency of the induced voltage corresponding to a four-pole, 60-Hz induction motor operating at a speed of 1740 r/min.

What is the corresponding slip?

3. A three-phase induction motor runs at almost 1198 r/min at no load and 1112 r/min at full load when supplied from a 60-Hz, three-phase source.

a. How many poles does this motor have?

b. What is the slip in percent at full load?

c. What is the corresponding frequency of the rotor currents?

d. What is the corresponding speed of the rotor field with respect to the rotor? With respect to the stator?

4. Linear induction motors have been proposed for a variety of applications including high-speed ground transportation. A linear motor based on the induction-motor principle consists of a car riding on a track. The track is a developed squirrel-cage winding, and the car, which is 4.5 m long and 1.25 m wide, has a developed three-phase, 12-pole-pair armature winding. Power at 75 Hz is fed to the car from arms extending through slots to rails below ground level.

a. What is the synchronous speed in km/hr?

b. Will the car reach this speed? Explain your answer.

c. What is the slip if the car is traveling 95 km/hr? What is the frequency of the track currents under this condition?

d. If the control system controls the magnitude and frequency of the car currents to maintain constant slip, what is the frequency of the armature-winding currents when the car is traveling 75 km/hr? What is the frequency of the track currents under this condition?

5. A three-phase, variable-speed induction motor is operated from a variable-frequency, variable-voltage source which is controlled to maintain constant peak air-gap flux density as the frequency of the applied voltage is varied. The motor is to be operated at constant slip frequency while the motor speed is varied between one half rated speed and rated speed.

a. Describe the variation of magnitude and frequency of the applied voltage with speed.

b. Describe how the magnitude and frequency of the rotor currents will vary as the motor speed is varied.

c. How will the motor torque vary with speed?

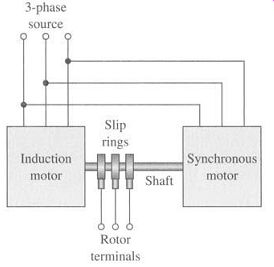
FIG. 23 Interconnected induction and synchronous machines (Problems 7 and 8).

6. Describe the effect on the torque-speed characteristic of an induction motor produced by (a) halving the applied voltage and (b) halving both the applied voltage and the frequency. Sketch the resultant torque-speed curves relative to that of rated-voltage and rated-frequency. Neglect the effects of stator resistance and leakage reactance.

7. FIG. 23 shows a system consisting of a three-phase wound-rotor induction machine whose shaft is rigidly coupled to the shaft of a three-phase synchronous motor. The terminals of the three-phase rotor winding of the induction machine are brought out to slip rings as shown. With the system supplied from a three-phase, 60-Hz source, the induction machine is driven by the synchronous motor at the proper speed and in the proper direction of rotation so that three-phase, 120-Hz voltages appear at the slip rings. The induction motor has four-pole stator winding.

a. How many poles are on the rotor winding of the induction motor?

b. If the stator field in the induction machine rotates in a clockwise direction, what is the rotation direction of its rotor?

c. What is the rotor speed in r/min?

d. How many poles are there on the synchronous motor?

e. It is proposed that this system can produce dc voltage by reversing two of the phase leads to the induction motor stator. Is this proposal valid?

8. A system such at that shown in FIG. 23 is used to convert balanced 50-Hz voltages to other frequencies. The synchronous motor has four poles and drives the interconnected shaft in the clockwise direction. The induction machine has six poles and its stator windings are connected to the source in such a fashion as to produce a counterclockwise rotating field (in the direction opposite to the rotation of the synchronous motor). The machine has a wound rotor whose terminals are brought out through slip rings.

a. At what speed does the motor run?

b. What is the frequency of the voltages produced at the slip rings of the induction motor?

c. What will be the frequency of the voltages produced at the slip rings of the induction motor if two leads of the induction-motor stator are interchanged, reversing the direction of rotation of the resultant rotating field?

9. A three-phase, eight-pole, 60-Hz, 4160-V, 1000-kW squirrel-cage induction motor has the following equivalent-circuit parameters in ohms per phase Y referred to the stator:

R1 = 0.220

R2 = 0.207

X1 = 1.95

X2 = 2.42

Xm = 45.7

Determine the changes in these constants which will result from the following proposed design modifications. Consider each modification separately.

a. Replace the stator winding with an otherwise identical winding with a wire size whose cross-sectional area is increased by 4 percent.

b. Decrease the inner diameter of the stator laminations such that the air gap is decreased by 15 percent.

c. Replace the aluminum rotor bars (conductivity 3.5 × 107 mhos/m) with copper bars (conductivity 5.8 × 107 mhos/m).

d. Reconnect the stator winding, originally connected in Y for 4160-V operation, in A for 2.4 kV operation.

10. A three-phase, Y-connected, 460-V (line-line), 25-kW, 60-Hz, four-pole induction motor has the following equivalent-circuit parameters in ohms per phase referred to the stator:

R1=0.103

R2=0.225

X1=I.10

X2=1.13

Xm=59.4

The total friction and windage losses may be assumed constant at 265 W, and the core loss may be assumed to be equal to 220 W. With the motor connected directly to a 460-V source, compute the speed, output shaft torque and power, input power and power factor and efficiency for slips of 1, 2 and 3 percent. You may choose either to represent the core loss by a resistance connected directly across the motor terminals or by resistance Rc connected in parallel with the magnetizing reactance Xm.

11. Consider the induction motor of Problem 10.

a. Find the motor speed in r/min corresponding to the rated shaft output power of 25 kW. (Hint: This can be easily done by writing a MATLAB script which searches over the motor slip.)

b. Similarly, find the speed in r/min at which the motor will operate with no external shaft load (assuming the motor load at that speed to consist only of the friction and windage losses).

c. Write a MATLAB script to plot motor efficiency versus output power as the motor output power varies from zero to full load.

d. Make a second plot of motor efficiency versus output power as the motor output power varies from roughly 5 kW to full load.

12 Write a MATLAB script to analyze the performance of a three-phase induction motor operating at its rated frequency and voltage. The inputs should be the rated motor voltage, power and frequency, the number of poles, the equivalent-circuit parameters, and the rotational loss. Given a specific speed, the program should calculate the motor output power, the input power and power factor and the motor efficiency. Exercise your program on a 500-kW, 4160 V, three-phase, 60-Hz, four-pole induction motor operating at 1725 r/min whose rated speed rotational loss is 3.5 kW and whose equivalent-circuit parameters are:

R1 =0.521

R2=1.32

X1 =4.98

X2=5.32

Xm=136

13. A 15-kW, 230-V, three-phase, Y-connected, 60-Hz, four-pole squirrel-cage induction motor develops full-load internal torque at a slip of 3.5 percent when operated at rated voltage and frequency. For the purposes of this problem, rotational and core losses can be neglected. The following motor parameters, in ohms per phase, have been obtained:

R1 = 0.21

X1 -X2 = 0.26

Xm = 10.1

Determine the maximum internal torque at rated voltage and frequency, the slip at maximum torque, and the internal starting torque at rated voltage and frequency.

14. The induction motor of Problem 13 is supplied from a 230-V source through a feeder of impedance Zf -0.05 + j0.14 ohms. Find the motor slip and terminal voltage when it is supplying rated load.

15. A three-phase induction motor, operating at rated voltage and frequency, has a starting torque of 135 percent and a maximum torque of 220 percent, both with respect to its rated-load torque. Neglecting the effects of stator resistance and rotational losses and assuming constant rotor resistance, determine:

a. the slip at maximum torque.

b. the slip at rated load.

c. the rotor current at starting (as a percentage of rotor current at rated load).

16. When operated at rated voltage and frequency, a three-phase squirrel-cage induction motor (of the design classification known as a high-slip motor) delivers full load at a slip of 8.7 percent and develops a maximum torque of 230 percent of full load at a slip of 55 percent. Neglect core and rotational losses and assume that the rotor resistance and inductance remain constant, independent of slip. Determine the torque at starting, with rated voltage and frequency, in per unit based upon its full-load value.

17. A 500-kW, 2400-V, four-pole, 60-Hz induction machine has the following equivalent-circuit parameters in ohms per phase Y referred to the stator: R1 = 0.122 R2 -0.317 X 1 = 1.364 X2 -1.32 Xm = 45.8

It achieves rated shaft output at a slip of 3.35 percent with an efficiency of 94.0 percent. The machine is to be used as a generator, driven by a wind turbine. It will be connected to a distribution system which can be represented by a 2400-V infinite bus.

a. From the given data calculate the total rotational and core losses at rated load.

b. With the wind turbine driving the induction machine at a slip of -3.2 percent, calculate (i) the electric power output in kW, (ii) the efficiency (electric power output per shaft input power) in percent and (iii) the power factor measured at the machine terminals.

c. The actual distribution system to which the generator is connected has an effective impedance of 0.18 + j0.41 f2/phase. For a slip of -3.2 percent, calculate the electric power as measured (i) at the infinite bus and (ii) at the machine terminals.

18. Write a MATLAB script to plot the efficiency as a function of electric power output for the induction generator of Problem 17 as the slip varies from -0.5 to -3.2 percent. Assume the generator to be operating into the system with the feeder impedance of part (c) of Problem 17.

19. For a 25-kW, 230-V, three-phase, 60-Hz squirrel-cage motor operating at rated voltage and frequency, the rotor I2R loss at maximum torque is 9.0 times that at full-load torque, and the slip at full-load torque is 0.023.

Stator resistance and rotational losses may be neglected and the rotor resistance and inductance assumed to be constant. Expressing torque in per unit of the full-load torque, find

a. the slip at maximum torque.

b. the maximum torque.

c. the starting torque.

20 A squirrel-cage induction motor runs at a full-load slip of 3.7 percent.

The rotor current at starting is 6.0 times the rotor current at full load. The rotor resistance and inductance is independent of rotor frequency and rotational losses, stray-load losses and stator resistance may be neglected.

Expressing torque in per unit of the full-load torque, compute

a. the starting torque.

b. the maximum torque and the slip at which the maximum torque occurs.

21. A A-connected, 25-kW, 230-V, three-phase, six-pole, 50-Hz squirrel-cage induction motor has the following equivalent-circuit parameters in ohms per phase Y: R1 = 0.045 R2 = 0.054 X1 = 0.29 X 2 = 0.28 Xm = 9.6

a. Calculate the starting current and torque for this motor connected directly to a 230-V source.

b. To limit the starting current, it is proposed to connect the stator winding in Y for starting and then to switch to the A connection for normal operation. (i) What are the equivalent-circuit parameters in ohms per phase for the Y connection? (ii) With the motor Y-connected and running directly off of a 230-V source, calculate the starting current and torque.

22. The following data apply to a 125-kW, 2300-V, three-phase, four pole, 60-Hz squirrel-cage induction motor: Stator-resistance between phase terminals = 2.23 ft No-load test at rated frequency and voltage: Line current = 7.7 A Three-phase power = 2870 W Blocked-rotor test at 15 Hz: Line voltage = 268 V Line current = 50.3 A Three-phase power = 18.2 kW

a. Calculate the rotational losses.

b. Calculate the equivalent-circuit parameters in ohms. Assume that X l =X 2.

c. Compute the stator current, input power and power factor, output power and efficiency when this motor is operating at rated voltage and frequency at a slip of 2.95 percent.

23. Two 50-kW, 440-V, three-phase, six-pole, 60-Hz squirrel-cage induction motors have identical stators. The dc resistance measured between any pair of stator terminals is 0.204 ft. Blocked-rotor tests at 60-Hz produce the following results:

Determine the ratio of the internal starting torque developed by motor 2 to that of motor 1 (a) for the same current and (b) for the same voltage. Make reasonable assumptions.

24. Write a MATLAB script to calculate the parameters of a three-phase induction motor from open-circuit and blocked-rotor tests.

Input: Rated frequency Open-circuit test: Voltage, current and power Blocked-rotor test: Frequency, voltage, current and power Stator-resistance measured phase to phase Assumed ratio X1 / X2

Output: Rotational loss Equivalent circuit parameters R1, R2, X1, X2 and X m Exercise your program on a 2300-V, three-phase, 50-Hz, 250-kW induction motor whose test results are: Stator-resistance between phase terminals = 0.636 g2 No-load test at rated frequency and voltage: Line current = 20.2 A Three-phase power -3.51 kW Blocked-rotor test at 12.5 Hz: Line voltage = 142 V Line current -62.8 A Three-phase power -6.55 kW You may assume that X1 = 0.4(Xl + X2).

25. A 230-V, three-phase, six-pole, 60-Hz squirrel-cage induction motor develops a maximum internal torquelof 288 percent at a slip of 15 percent when operated at rated voltage and frequency. If the effect of stator resistance is neglected, determine the maximum internal torque that this motor would develop if it were operated at 190 V and 50 Hz. Under these conditions, at what speed would the maximum torque be developed?

26. A 75-kW, 50-Hz, four-pole, 460-V three-phase, wound-rotor induction motor develops full-load torque at 1438 r/min with the rotor short-circuited. An external non-inductive resistance of 1.1 ~ is placed in series with each phase of the rotor, and the motor is observed to develop its rated torque at a speed of 1405 r/min. Calculate the rotor resistance per phase of the motor itself.

27. A 75-kW, 460-V, three-phase, four-pole, 60-Hz, wound-rotor induction motor develops a maximum internal torque of 225 percent at a slip of 16 percent when operated at rated voltage and frequency with its rotor short-circuited directly at the slip rings. Stator resistance and rotational losses may be neglected, and the rotor resistance and inductance may be assumed to be constant, independent of rotor frequency. Determine

a. the slip at full load in percent.

b. the rotor IZR loss at full load in watts.

c. the starting torque at rated voltage and frequency in per unit and in N. m.

If the rotor resistance is doubled (by inserting external series resistance at the slip rings) and the motor load is adjusted for such that the line current is equal to the value corresponding to rated load with no external resistance, determine

d. the corresponding slip in percent and

e. the torque in N. m.

28. Neglecting any effects of rotational and core losses, use MATLAB to plot the internal torque versus speed curve for the induction motor of Problem 10 for rated-voltage, rated-frequency operation. On the same plot, plot curves of internal torque versus speed for this motor assuming the rotor resistance increases by a factor of 2, 5 and 10.

29. A 100-kW, three-phase, 60-Hz, 460-V, six-pole wound-rotor induction motor develops its rated full-load output at a speed of 1158 r/min when operated at rated voltage and frequency with its slip rings short-circuited. The maximum torque it can develop at rated voltage and frequency is 310 percent of full-load torque. The resistance of the rotor winding is 0.17 S2/phase Y. Neglect any effects of rotational and stray-load loss and stator resistance.

a. Compute the rotor I^2R loss at full load.

b. Compute the speed at maximum torque in r/min.

c. How much resistance must be inserted in series with the rotor windings to produce maximum starting torque? With the rotor windings short-circuited, the motor is now run from a 50-Hz supply with the applied voltage adjusted so that the air-gap flux wave is essentially equal to that at rated 60-Hz operation.

d. Compute the 50-Hz applied voltage.

e. Compute the speed at which the motor will develop a torque equal to its rated 60-Hz value with its slip-tings shorted.

30. A 460-V, three-phase, six-pole, 60-Hz, 150-kW, wound-rotor induction motor develops an internal torque of 190 percent with a line current of 200 percent (torque and current expressed as a percentage of their full-load values) at a slip of 5.6 percent when running at rated voltage and frequency with its rotor terminals short-circuited. The rotor resistance is measured to be 90 mfl between each slip ring and may be assumed to remain constant. A balanced set of Y-connected resistors is to be connected to the slip rings in order to limit the rated-voltage starting current to 200 percent of its rated value. What resistance must be chosen for each leg of the Y connection? What will be the starting torque under these conditions?

31. The resistance measured between each pair of slip rings of a three-phase, 60-Hz, 250-kW, 16-pole, wound-rotor induction motor is 49 mfl. With the slip tings short-circuited, the full-load slip is 0.041. For the purposes of this problem, it may be assumed that the slip-torque curve is a straight line from no load to full load. The motor drives a fan which requires 250 kW at the full-load speed of the motor. Assuming the torque to drive the fan varies as the square of the fan speed, what resistance should be connected in series with the rotor resistance to reduce the fan speed to 400 ffmin?