Power Systems--Three-Phase Electric Power Systems

1. Case for Balanced Polyphase Power Systems

Electric power is transmitted from source to load in the circuit shown in FIG. 1a, and is proportional to the root mean square (RMS) voltage between conductors (V) and the RMS current (I). There are certain voltages determined by IEEE/ANSI standards from which one may choose. The selection is made for a given application based on several factors, including availability, cost, power level, and safety. Given that the voltage has been selected, the transmitted power is proportional to current; hence, the more power to be delivered to the load, the greater the current. The upper limit on current-carrying capacity (i.e., ampacity) for a conductor is proportional to the cross-sectional area (A), as is its cost. So, for a given load, we need "2A worth" of conductors, where A is the requisite cross-sectional area required to carry the current to and from the load.

Now suppose the load triples. We modify the system by using three sources, serving one-third the total load from three identical circuits, as shown in FIG. 1b. Even though we have tripled the capacity, we have also tripled the conductor cost, since we now need a total of "6A" conductors. We further modify the system by making a common return path, as shown in FIG. 1c. Apparently, this accomplishes little since the return conductor must have size 3A since it must have three times the ampacity, and we still need a total "6A" worth of conductors.

But suppose we modified the source so that three voltages, equal in magnitude, and 120° phase displaced, were used. Analysis shows that the currents in conductors a, b, and c are equal, and the return current (n) is zero!. Hence, we need only "3A worth" of conductor to serve the same load as in FIG. 1b, a 50% savings! These dramatic savings are only fully realized for the perfectly balanced case, and most systems install a neutral to accommodate load imbalance. However, experience shows that even for worse-case imbalance, n usually carries no more current than a, b, or c, and hence need be no larger than A. Even so, this represents a significant 33% savings and, frequently, conductor n is sized even smaller. These same benefits accrue to any polyphase system; the reason "three" is usually chosen is because it results in economical apparatus (transformer, generator, motor, transmission line) design.

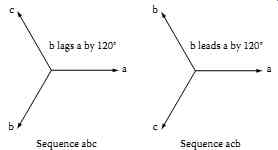
We pause to define some terms. Let the conductors a, b, and c be referred to as the "phases" and conductor n as the neutral. The arrangement of FIG. 1c, shall be called "three-phase." It is fundamental to three-phase systems that the voltages be equal in magnitude, and 120° phase displaced, or nearly so.

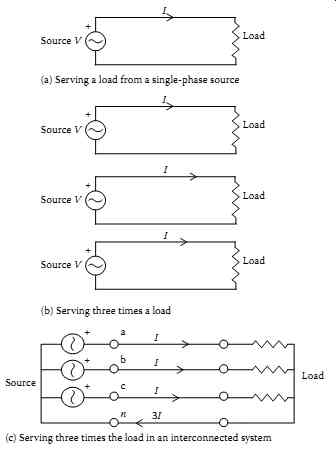
FIG. 1 Basic single-phase power delivery schemes. (a) An electric power delivery circuit. (b) A modified system with triple capacity. (c) System (b) with a common return conductor.

2. Balanced Three-Phase Circuit Analysis

Consider FIG. 2, which is fundamental to our three-phase discussion.

For balanced operation the voltages are defined as ...

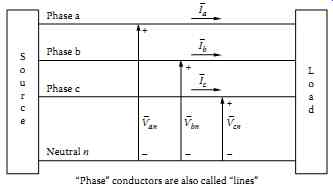
FIG. 2 The basic three-phase situation.

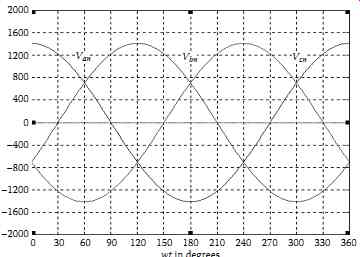
FIG. 3 The instantaneous phase-to-neutral voltages.

FIG. 4

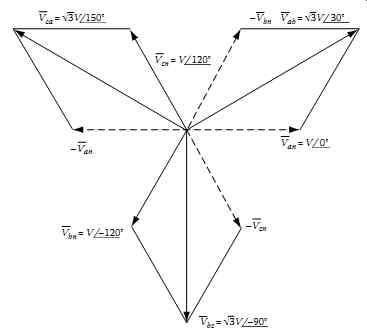
FIG. 5 The voltage phasor diagram for a balanced three-phase system.

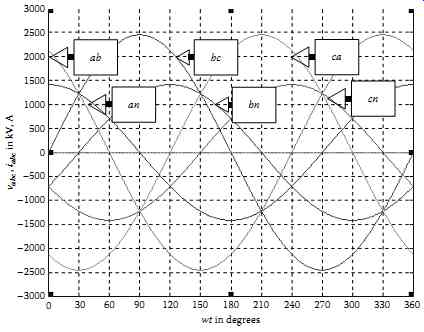
FIG. 6 The instantaneous voltages in a balanced three-phase system.

2.1 Wye and Delta Connections

The currents in the system will be determined by the load impedances. If the currents (a, b, c) are to be balanced (i.e., equal in magnitude; 120° phase displaced), the impedances must meet two conditions:

• The three-phase connection must be symmetrical.

• The three phase impedances must be equal.

The two connections that meet the first criteria are shown in FIG. 7.

Note that in the wye connection, the neutral can be "brought out" and connected into a four-wire system, whereas in the delta connection, the neutral always leads to an open circuit.

The currents in the wye case are ...

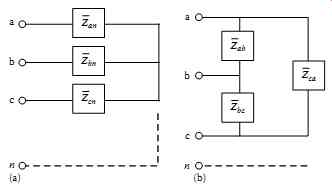
FIG. 7 Balanced three-phase connections. (a) The wye (Y) connection and (b) the delta (Δ) connection.

FIG. 8

FIG. 9

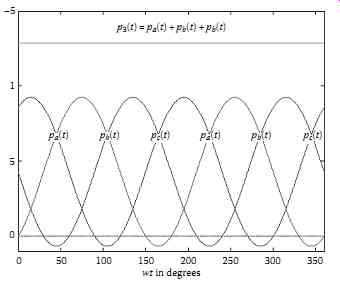
FIG. 10 Instantaneous power in a balanced three-phase situation.

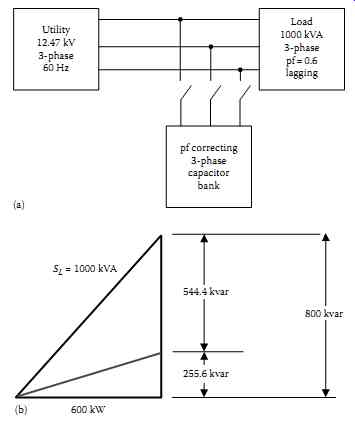
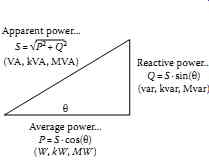
FIG. 11 Power factor correction. (a) System components and (b) complex powers in P-Q coordinates.

4. Summary

Three-phase concepts are commonly encountered in power systems, where power levels exceed 10 kW.

Such systems are designed to operate as balanced, meaning equal in magnitude, and 120° phase displaced, when applied to voltages and currents, and equal, when applied to impedance and power.

Some advantages of balanced three-phase operation are

1. Transmission of twice the power using a given amount of conductor, compared with the single-phase case.

2. DC transmission of instantaneous power, compared with the oscillatory nature of single-phase power transmission.

3. Choice of two voltage levels.

4. Some transmission capability remains, even with the loss of two phases.

For analysis of balanced three-phase systems, symmetry can be used to great advantage, eliminating two-thirds of the work, making calculations not much more complicated that those for the single-phase case. Unbalanced operation is considered an abnormality, and is best analyzed using symmetrical components.