AMAZON multi-meters discounts AMAZON oscilloscope discounts
.
Voltage flicker is a problem that has existed in the power industry for many years. Many types of end-use equipment can create voltage flicker, and many types of solution methods are available. Fortunately, the problem is not overly complex, and it can often be analyzed using fairly simple methods. In many cases, however, solutions can be expensive. Perhaps the most difficult aspect of the voltage flicker problem has been the development of a widely accepted definition of just what "flicker" is and how it can be quantified in terms of measurable quantities.
To electric utility engineers, voltage flicker is considered in terms of magnitude and rate of change of voltage fluctuations. To the utility customer, however, flicker is considered in terms of "my lights are flickering." The necessary presence of a human observer to "see" the change in lamp (intensity) output in response to a change in supply voltage is the most complex factor for which to account. Significant research, dating back to the early twentieth century, has been devoted to establishing an accurate correlation between voltage changes and observer perceptions. This correlation is essential so that a readily measurable quantity, supply voltage, can be used to predict a human response.
The early work regarding voltage flicker considered voltage flicker to be a single-frequency modulation of the power frequency voltage. Both sinusoidal and square wave modulations were considered as shown mathematically in Equations 1 and 2, with most work concentrating on square wave modulation.
(eq.1)
(eq.2)
Based on Equations 1 and 2, the voltage flicker magnitude can be expressed as a percentage of the root-mean-square (rms) voltage, where the term "V" in the two equations represents the percentage.
While both the magnitude of the fluctuations ("V") and the "shape" of the modulating waveform are obviously important, the frequency of the modulation is also extremely relevant and is explicitly rep resented as _m. For sinusoidal flicker (given by Equation 1), the total waveform appears as shown in FGR. 1 with the modulating waveform shown explicitly. A similar waveform can be easily created for square-wave modulation.
To correlate the voltage change percentage, V, at a certain frequency, _m, with human perceptions, early research led to the widespread use of what is known as a flicker curve to predict possible observer complaints. Flicker curves are still in widespread use, particularly in the United States. A typical flicker curve is shown in FGR. 2 and is based on tests conducted by the General Electric Company. It’s important to realize that these curves are developed based on square wave modulation. Voltage changes from one level to another are considered to be "instantaneous" in nature, which may or may not be an accurate representation of actual equipment-produced voltage fluctuations.
The curve of FGR. 2 requires some explanation in order to understand its application. The "threshold of visibility" corresponds to certain fluctuation magnitude and frequency pairs that rep resent the borderline above which an observer can just perceive lamp (intensity) output variations in a 120 V, 60 Hz, 60 W incandescent bulb. The "threshold of irritation" corresponds to certain fluctuation magnitude and frequency pairs that represent the borderline above which the majority of observers would be irritated by lamp (intensity) output variations for the same lamp type. Two conclusions are immediately apparent from these two curves: (1) even small percentage changes in supply voltage can be noticed by persons observing lamp output, and (2) the frequency of the voltage fluctuations is an important consideration, with the frequency range from 6 to 10 Hz being the most sensitive.
Most utility companies don’t permit excessive voltage fluctuations on their system, regardless of the frequency. For this reason, a "typical" utility flicker curve will follow either the "threshold of irritation" or the "threshold of visibility" curve as long as the chosen curve lies below some established value (2% in FGR. 2). By requiring that voltage fluctuations not exceed the "borderline of visibility" curve, the utility is insuring conservative criteria that should minimize potential problems due to volt age fluctuations.
===
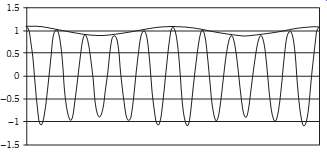
FGR. 1 Sinusoidal voltage flicker.
===
To other customers, Zxfmr, Zsource, Vsource, Fluctuating load
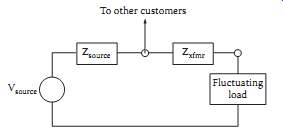
FGR. 3 Example circuit for flicker calculations.
===
- Threshold of irritation
- Threshold of visibility
- "Typical" flicker curve
- Fluctuations per hour
- Fluctuations per minute
- Fluctuations per second
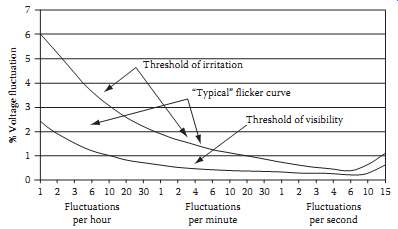
FGR. 2 Typical flicker curves.
===
For many years, the generic flicker curve has served the utility industry well. Fluctuating motor loads like car shredders, wood chippers, and many others can be fairly well characterized in terms of a duty cycle and a maximum torque. From this information, engineers can predict the magnitude and frequency of voltage changes anywhere in the supplying transmission and distribution system. Voltage fluctuations associated with motor starting events are also easily translated into a point (or points) on the flicker curve, and many utilities have based their motor starting criteria on this method for many years. Other loads, most notably arcing loads, cannot be represented as a single flicker magnitude and frequency term. For these types of loads, utility engineers typically presume either worst-case or most likely variations for analytical evaluations.
Regardless of the type of load, the typical calculation procedure involves either basic load flow or simple voltage division calculations. FGR. 3 shows an example positive sequence circuit with all data assumed in per-unit on consistent bases.
For fluctuating loads that are best represented by a constant power model (arc furnaces and load torque variations on a running motor), basic load flow techniques can be used to determine the full-load and no-load (or "normal condition") voltages at the "critical" or "point of common coupling" bus where other customers might be served. For fluctuating loads that are best represented by a constant impedance model (motor starting), basic circuit analysis techniques readily provide the full-load and no-load ("normal condition") voltages at the critical bus. Regardless of the modeling and calculation procedures used, equations similar to Equation 3 can be used to determine the percentage voltage change for use in conjunction with a flicker curve. Of course, accurate information regarding the frequency of the assumed fluctuation is absolutely necessary. Note that Equation 3 represents an over-simplification and should therefore not be used in cases where the fluctuations are frequent enough to impact the average rms value (measured over several seconds up to a minute). Other more elaborate formulas are available for these situations.
%Voltage change = (3)
From a utility engineer's viewpoint, the decision to either serve or deny service to a fluctuating load is often based on the result of Equation 3 (or a more complex version of Equation 3) including information about the frequency at which the calculated change occurs. From this simplified discussion, several questions arise:
1. How are fluctuating loads taken into account when the nature of the fluctuations is not constant in magnitude?
2. How are fluctuating loads taken into account when the nature of the fluctuations is not constant in frequency?
3. How are static compensators and other high response speed mitigation devices included in the calculations?
===
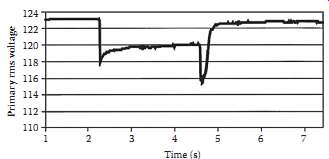
FGR. 4 Poorly timed motor starter voltage fluctuation.
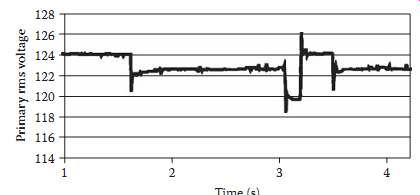
FGR. 5 Adaptive-var compensator effects.
===
Input transformer Block 1 Block 2 dB 1 -3
0.05 0 8.8 Range selector
0.5 1.0 2.0 5.0 10.0 20.0
%
?V V Hz Weighting filters 35 Hz Block 3 Block 4 Block 5 A/D converter sampling rate =50 Hz
Squaring multiplier Squaring and smoothing Square rooter ? - v 1 min integrator Statistical evaluation of flicker level Programming of short and long observation periods Output and data display and recording Output 5 recording Output 4 short time integration Output 3 range selection Output 2 weighted voltage fluctuation 64 level classifier Output interfaces 1st order sliding mean filter Simulation of lamp-eye brain response Detector and gain control Demodulator with squaring multiplier Signal generator for calibration checking Input voltage adaptor rms Meter Output 1 half cycle rms voltage indication
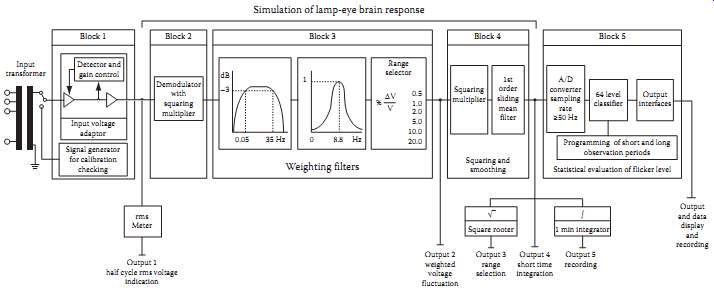
FGR. 6 Flicker meter block diagram.
===
Changes (min)
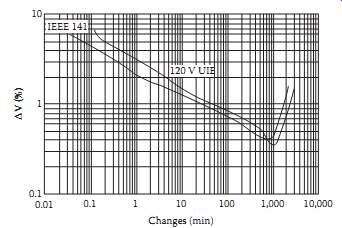
FGR. 7 Threshold of irritation flicker curve and Pst = 1.0 curve from a flicker
meter.
===
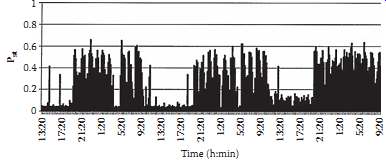
FGR. 8 Short-term flicker severity example plot.
===
As examples, consider the rms voltage plots (on 120 V bases) shown in FGR. 4 and 5. FGR. 4 shows an rms plot associated with a poorly timed two-step reduced-voltage motor starter. FGR. 5 shows a motor starting event when the motor is compensated by an adaptive-var compensator. Questions 1-3 are clearly difficult to answer for these plots, so it would be very difficult to apply the basic flicker curve.
In many cases of practical interest, "rules of thumb" are often used to answer approximately these and other related questions so that the simple flicker curve can be used effectively. However, these assumptions and approaches must be conservative in nature and may result in costly equipment modifications prior to connection of certain fluctuating loads. In modern environment, it’s imperative that end-users operate at the least total cost. It’s equally important that end-use fluctuating loads not create problems for other users. Due to the conservative and approximate nature of the flicker curve methodology, there is often significant room for negotiation, and the matter is often not settled considering only engineering results.
For roughly three decades, certain engineering groups have recognized the limitations of the flicker curve methods and have developed alternative approaches based on an instrument called a flicker meter. This work, driven strongly in Europe by the International Union for Electroheat (UIE) and the International Electrotechnical Commission (IEC), appears to offer solutions to many of the problems with the flicker curve methodology. Many years of industrial experience have been obtained with the flicker meter approach, and its output has been well-correlated with complaints of utility customers. At this time, the Institute of Electrical and Electronics Engineers (IEEE) is working toward adopting the flicker meter methodology for use in North America.
The flicker meter is a continuous time measuring system that takes voltage as an input and produces three output indices that are related to customer perception. These outputs are: (1) instantaneous flicker sensation, Pinst, (2) short-term flicker severity, Pst, and (3) long-term flicker severity, Plt. A block diagram of an analog flicker meter is shown in FGR. 6.
The flicker meter takes into account both the physical aspects of engineering (how does the lamp
[intensity] output vary with voltage?) and the physiological aspects of human observers (how fast can the human eye respond to light changes?). Each of the five basic blocks in FGR. 6 contribute to one or both of these aspects. While a detailed discussion of the flicker meter is beyond the scope of this section, the function of the blocks can be summarized as follows.
Blocks 1 and 2 act to process the input voltage signal and to partially isolate only the modulating term in Equations 1 or 2. Block 3 completes the isolation of the modulating signal through complex filtering and applies frequency-sensitive weighting to the "pure" modulating signal. Block 4 models the physiological response of the human observer, specifically the short-term memory tendency of the brain to correlate the voltage modulating signal with a human perception ability. Block 5 performs statistical analysis on the output of Block 4 to capture the cumulative effects of fluctuations over time.
The instantaneous flicker sensation is the output of Block 4. The short- and long-term severity indices are the outputs of Block 5. Pinst is available as an output quantity on a continuous basis, and a value of 1.0 corresponds with the threshold of visibility curve in FGR. 2. A single Pst value is available as an output every 10 min, and a value of 1.0 corresponds to the threshold of irritation curve in FGR. 2. Of course, a comparison can only be made for certain inputs.
For square wave modulation, FGR. 7 shows a comparison of the "irritation level" given by IEEE Std. 141 (Red Book) and that level predicted by the flicker meter to be "irritating" (Pst = 1.0). For these comparisons, the lamp type used is a 120 V, 60 Hz, 60 W incandescent bulb. Note that the flicker curve taken from IEEE Std. 141 is essentially identical to the "borderline of irritation" curve given in FGR. 2.
As FGR. 7 clearly demonstrates, the square wave modulation voltage fluctuations that lead to irritation are nearly identical as predicted by either a standard flicker curve or a flicker meter.
The real advantage of the flicker meter methodology lies in that fact that the continuous time measurement system can easily predict possible irritation for arbitrarily complex modulation waveforms.
As an example, FGR. 8 shows a plot of Pst over a 3-day period at a location serving a small electric arc furnace. (Note: In this case, there were no reported customer complaints and Pst was well below the irritation threshold value of 1.0 during the entire monitoring period.) Due to the very random nature of the fluctuations associated with an arc furnace, the flicker curve methodology cannot be used directly as an accurate predictor of irritation levels because it’s appropriate only for the "sudden" voltage fluctuations associated with square wave modulation. The trade-off required for more accurate flicker prediction, however, is that the inherent simplicity of the basic flicker curve is lost.
For the basic flicker curve, simple calculations based on circuit and equipment models in FGR. 3 can be used. Data for these models is readily available, and time-tested assumptions are widely known for cases when exact data are not available. Because the flicker meter is a continuous-time system, continuous-time voltage input data is required for its use. For existing fluctuating loads, it’s reasonable to presume that a flicker meter can be connected and used to predict whether or not the fluctuations are irritating. However, it’s necessary to be able to predict potential flicker problems prior to the connection of a fluctuating load well before it’s possible to measure anything.
There are three possible solutions to the apparent "prediction" dilemma associated with the flicker meter approach. The most basic approach is to locate an existing fluctuating load that is similar to the one under consideration and simply measure the flicker produced by the existing load. Of course, the engineer is responsible for making sure that the existing installation is nearly identical to the one pro posed. While the fluctuating load equipment itself might be identical, supply system characteristics will almost never be the same.
Because the short-term flicker severity output of the f licker meter, Pst , is linearly dependent on voltage fluctuation magnitude over a wide range, it’s possible to linearly scale the Pst measurements from one location to predict those at another location where the supply impedance is different. (In most cases, voltage fluctuations are directly related to the supply impedance; a system with 10% higher supply impedance would expect 10% greater voltage fluctuation for the same load change.) In evaluations where it’s not possible to measure another existing fluctuating load, other approaches must be used.
If detailed system and load data are known, a time-domain simulation can be used to generate a continuous-time series of voltage data points. These points could then be used as inputs to a simulated flicker meter to predict the short-term flicker severity, Pst. This approach, however, is usually too intensive and time-consuming to be appropriate for most applications. For these situations, "shape factors" have been proposed that predict a Pst value for various types of fluctuations.
Shape factors are simple curves that can be used to predict, without simulation or measurement, the Pst that would be measured if the load were connected. Different curves exist for different "shapes" of voltage variation. Curves exist for simple square and triangular variations, as well as for more complex variations such as motor starting. To use a shape factor, an engineer must have some knowledge of (1) the magnitude of the fluctuation, (2) the shape of the fluctuation, including the time spent at each voltage level if the shape is complex, (3) rise time and fall times between voltage levels, and (4) the rate at which the shape repeats. In some cases, this level of data is not available, and assumptions are often made (on the conservative side). It’s interesting to note that the extreme of the conservative choices is a rectangular fluctuation at a known frequency; which is exactly the data required to use the basic flicker curve of FGR. 2.
Using either the flicker curve for simple evaluations or the flicker meter methodology for more complex evaluations, it’s possible to predict if a given fluctuating load will produce complaints from other customers. In the event that complaints are predicted, modifications must be made prior to granting service. The possible modifications can be made either on the utility side or on the customer (load) side (or both), or some type of compensation equipment can be installed.
In most cases, the most effective, but not least cost, ways to reduce or eliminate flicker complaints are to either (1) reduce the supply system impedance of the whole path from source to fluctuating load or (2) serve the fluctuating load from a dedicated and electrically remote (from other customers) circuit. In most cases, utility revenue projections for customers with fluctuating loads don’t justify such expenses, and the burden of mitigation is shifted to the consumer.
Customers with fluctuating load equipment have two main options regarding voltage flicker mitigation. In some cases, the load can be adjusted to the point that the frequency(ies) of the fluctuations are such that complaints are eliminated (recall the frequency-sensitive nature of the entire flicker problem). In other cases, direct voltage compensation can be achieved through high-speed static compensators.
Either thyristor-switched capacitor banks (often called adaptive var compensators or AVCs) or fixed capacitors in parallel with thyristor-switched reactors (often called static var compensators or SVCs) can be used to provide voltage support through reactive compensation in about one cycle. For loads where the main contributor to a large voltage fluctuation is a large reactive power change, reactive compensators can significantly reduce or eliminate the potential for flicker complaints. In cases where voltage fluctuations are due to large real power changes, reactive compensation offers only small improvements and can, in some cases, make the problem worse.
In conclusion, it’s almost always necessary to measure/predict flicker levels under a variety of possible conditions, both with and without mitigation equipment and procedures in effect. In very simple cases, a basic flicker curve will provide acceptable results. In more complex cases, however, an intensive measurement, modeling, and simulation effort may be required in order to minimize potential flicker complaints.
While this section has addressed the basic issues associated with voltage flicker complaints, prediction, and measurement, it’s not intended to be all-inclusive. A number of relevant publications, papers, reports, and standards are given for further reading, and the reader should certainly consider these documents carefully in addition to what is provided here.